Numbers can be written in different forms, and one of the most useful skills in math is knowing how to switch between decimals, fractions, and mixed numbers. Let’s explore how this works and why it’s important!
A rational number is any number that can be written as a fraction, where the numerator and denominator are both integers, and the denominator is not zero.
An irrational number cannot be written as a fraction and has a decimal expansion that goes on forever without repeating (e.g., π or √2).
Rational numbers either have terminating decimals (e.g., 0.5 = ½) or repeating decimals (e.g., 0.333... = ⅓).
Irrational numbers have decimals that go on forever without repeating, making them impossible to convert into exact fractions.
Repeating decimals are actually fractions in disguise! Here’s how to convert a repeating decimal into a fraction:
Example: Convert 0.727272... into a fraction
Let x be the repeating decimal:
x=0.727272...
Multiply by a power of 10 to shift the decimal:
100x=72.727272...
Subtract the original equation:
100x−x=72.727272...−0.727272...
99x=72
Solve for x:
𝑥=72/99
Simplify:
𝑥=8/11
So, 0.727272... = 8/11 as a fraction!
For terminating decimals, conversion is even easier!
Example: Convert 2.75 into a fraction
Write the decimal as a fraction:
2.75 = 275 / 100
Simplify:
275 / 100 = 11 / 4
Convert to a mixed number:
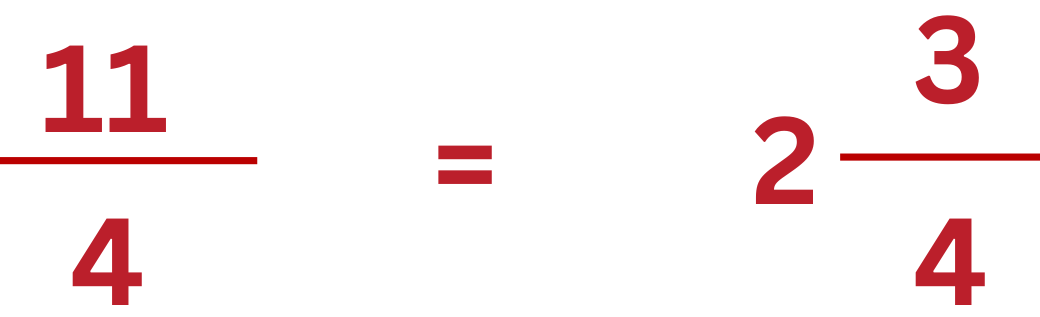
So, 2.75 = 2 ¾ as a mixed number!
Converting Fractions or Mixed Numbers to Decimals
To convert a fraction into a decimal, simply divide the numerator by the denominator.
Example: Convert ⅝ into a decimal
5÷8=0.625
So, ⅝ = 0.625
For mixed numbers, convert the fraction part separately and add it to the whole number.
Example:
Convert 3 ⅖ into a decimal
Convert ⅖ into a decimal:
2÷5=0.4
Add to the whole number: 3 + 0.4 = 3.4
So, 3 ⅖ = 3.4
Mastering these conversions helps in real-world applications like money, measurements, and problem-solving.
Let’s explore and practice conversion of decimals,fractions and mixed numbers with the help of NumericWiz.
For a limited time
