Ratios are everywhere! Whether you're adjusting a recipe, comparing prices, or analyzing speed, understanding equivalent ratios helps you make better decisions. But how do we know if two ratios are actually equivalent? There are three key methods to check: common factors, scaling, and direct comparison.
One way to check if two ratios are equivalent is to simplify them by dividing both terms by their greatest common factor (GCF). If they reduce to the same value, they are equivalent!
Example: Are 18:24 and 3:4 equivalent?
Find the GCF of 18 and 24, which is 6.
Divide both numbers by 6: 18/24=3/4
Since both ratios simplify to 3:4, they are equivalent!
Another way to check is by scaling up or down using multiplication or division. If you can multiply or divide both terms of one ratio by the same number to get the other ratio, they are equivalent.
Example: Is 5:8 equivalent to 25:40?
Multiply both terms of 5:8 by 5:
(5×5):(8×5)=25:40
Since we get 25:40, the ratios are equivalent!
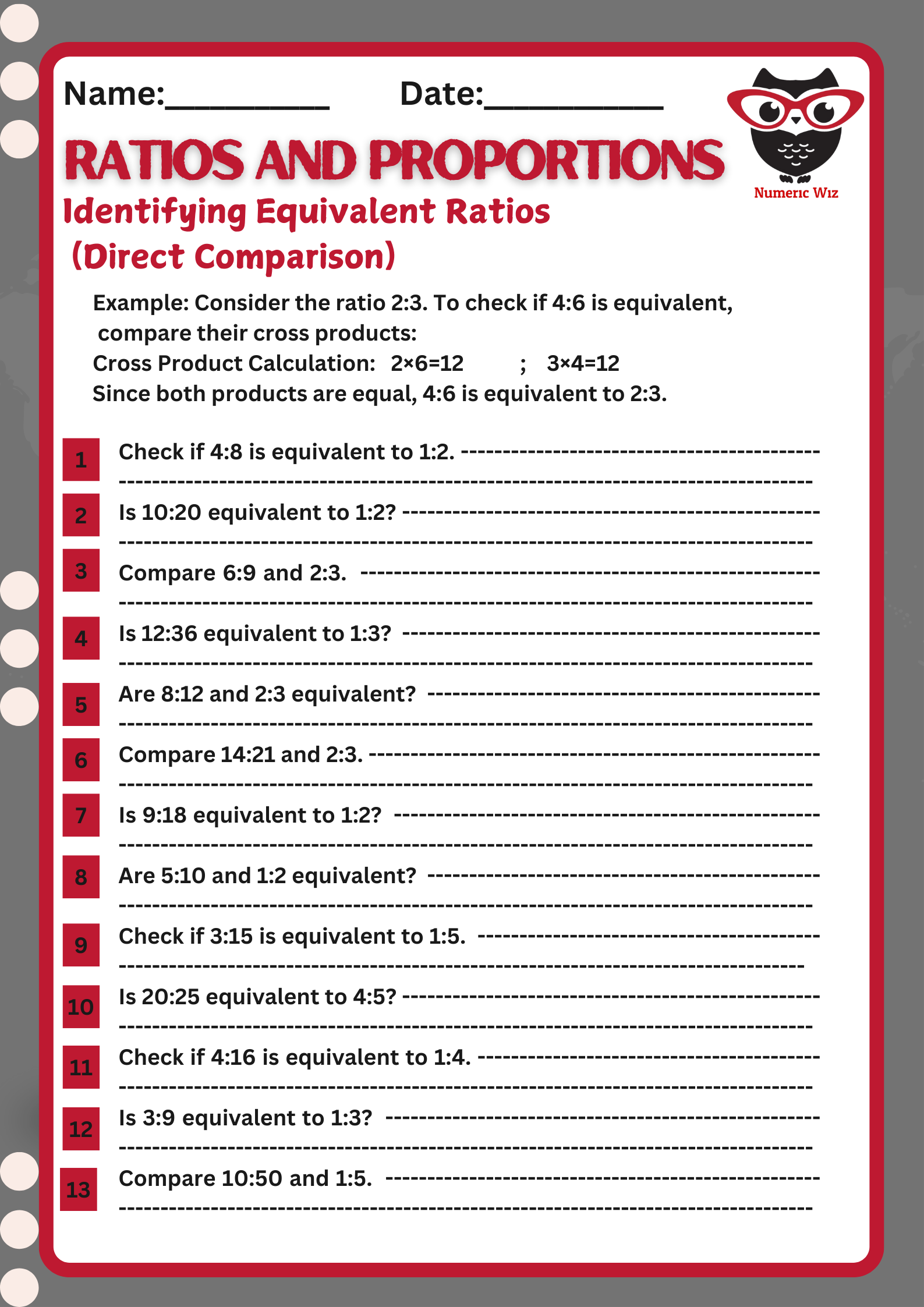
Direct Comparison
If the ratios are given as fractions, we can cross multiply to check equivalency. If the cross products are equal, the ratios are equivalent.
Example: Are 6:9 and 10:15 equivalent?
Write as fractions: 6/9 and 10/15
Cross multiply:
6×15=90,9×10=90
Since both products are equal (90 = 90), the ratios are equivalent!
✔ Helps compare prices and deals (e.g., Is 3 apples for $2 the same deal as 9 apples for $6?)
✔ Essential for proportions in math & science (e.g., mixing chemicals, scaling blueprints)
✔ Forms the foundation for understanding proportional relationships in graphs & tables
Cooking & Recipes – Adjust ingredient amounts while keeping the same taste (e.g., doubling a recipe).
Shopping & Discounts – Compare unit prices to find the best deal (e.g., 4 cookies for $2 vs. 12 cookies for $6).
Speed & Travel – Determine if two vehicles travel at the same speed (e.g., 150 miles in 3 hours vs. 250 miles in 5 hours).
Construction & Scale Models – Architects use ratios to design miniatures of buildings (e.g., a 500-ft skyscraper scaled down at 1:100).
For a limited time
