Understanding Unit Rates with Ratios of Quantities (Same or Different Units)

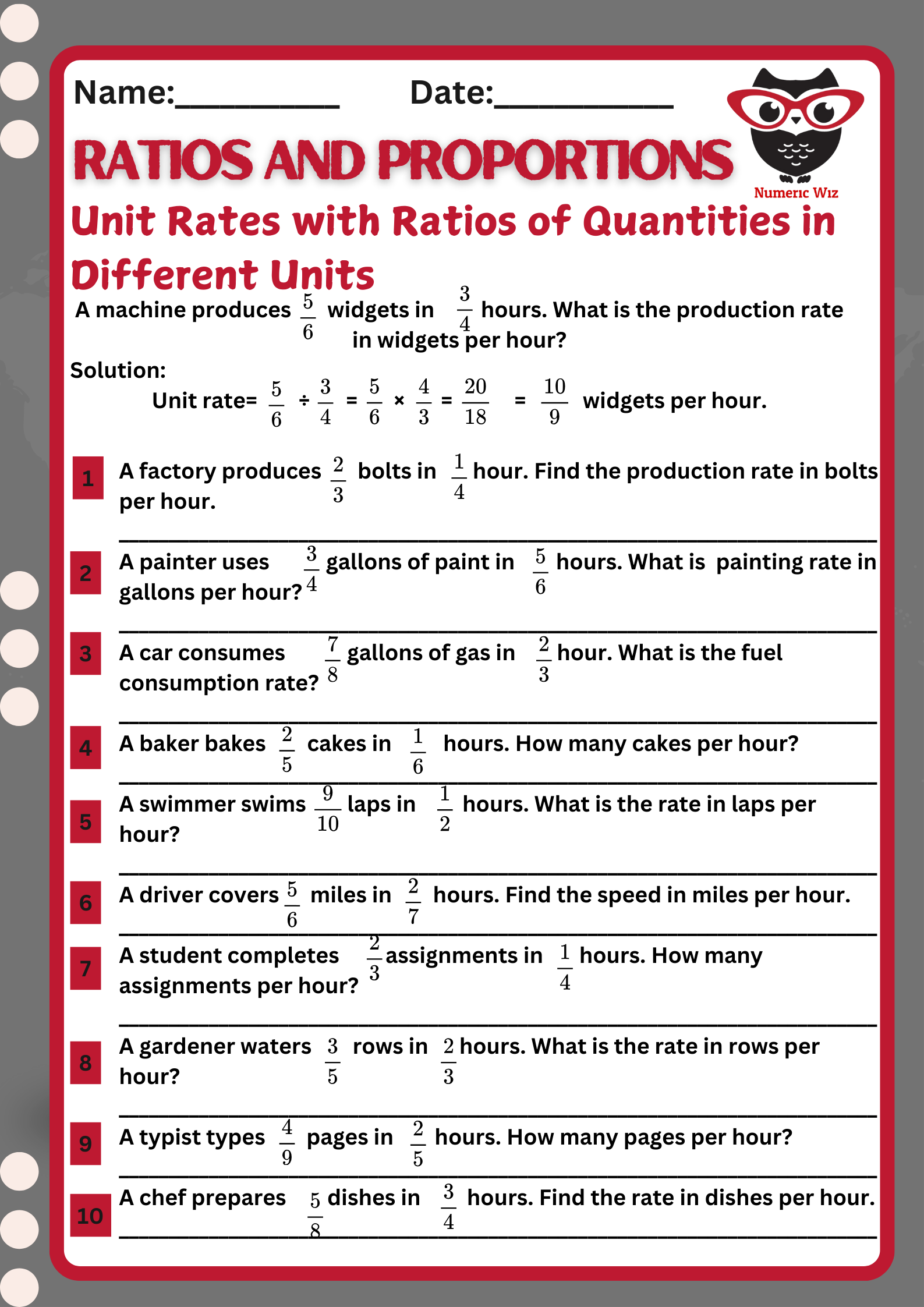
Unit rates help us compare different quantities by expressing how much one unit of a particular measure corresponds to another. When dealing with ratios of fractions, we often compute unit rates for lengths, areas, volumes, costs, speed, and more—whether the units are the same or different.

Unit Rates with the Same Units

When the numerator and denominator of a ratio have the same type of units, the unit rate is a pure number.

For example:

A ribbon is 3/4 meters long, and another is 1/2 meters long. The ratio of their lengths is:

3/4 ÷ 1/2 = 3/4 × 2/1 = 6/4 = 3/2

This means the first ribbon is 1.5 times the length of the second ribbon.

Unit Rates with Different Units

When the numerator and denominator have different units, the unit rate expresses a relationship between those units.

For example:

A car travels 5/2 miles in 3/4 hours. The speed (miles per hour) is:

5/2 ÷ 3/4 = 5/2 × 4/3 = 20/6 =10/3

So the car moves at 10/3 miles per hour (≈ 3.33 mph).

Steps to Compute Unit Rates

Set up the ratio based on the given fractions.

Divide the fractions by multiplying by the reciprocal.

Simplify the result to get the unit rate.

Interpret the unit rate in real-world terms.

Why Unit Rates with Ratios of Quantities Matter

✔ Compare different values in business, travel, engineering, and science.

✔ Measure efficiency (e.g., fuel consumption, productivity per hour).

✔ Make informed decisions based on cost-effectiveness, speed, or other factors.

Let's explore how unit rates help us compare different quantities in real-world situations!